Lesson
Challenge
Standards

Platonic Solids

What is it?

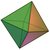
50px-Tetrahedron.jpg50px-Hexahedron.jpg50px-Dodecahedron.jpg50px-Octahedron.jpg50px-Icosahedron.jpg
The Platonic solids, or regular solids or regular polyhedra, are convex polyhedra with equivalent faces composed of congruent convex regular polygons. There are exactly five such solids (Steinhaus 1999, pp. 252-256): the cube, dodecahedron, icosahedron, octahedron, and tetrahedron, as was proved by Euclid in the last proposition of the Elements. The Platonic solids are sometimes also called cosmic figures (Cromwell 1997), although this term is sometimes used to refer collectively to both the Platonic solids and Kepler-Poinsot solids (Coxeter 1973).

The Platonic solids were known to the ancient Greeks, and were described by Plato in his Timaeus ca. 350 BC. In this work, Plato equated the tetrahedron with the element fire, the cube with earth, the icosahedron with water, the octahedron with air, and the dodecahedron with the stuff of which the constellations and heavens were made (Cromwell 1997). Predating Plato, the neolithic people of Scotland developed the five solids a thousand years earlier. The stone models are kept in the Ashmolean Museum in Oxford (Atiyah and Sutcliffe 2003).

Schläfli (1852) proved that there are exactly six regular bodies with Platonic properties (i.e., regular polytopes) in four dimensions, three in five dimensions, and three in all higher dimensions. However, his work (which contained no illustrations) remained practically unknown until it was partially published in English by Cayley (Schläfli 1858, 1860). Other mathematicians such as Stringham subsequently discovered similar results independently in 1880 and Schläfli's work was published posthumously in its entirety in 1901.

From www.mathsisfun.com

Why are there are only 5 Platonic Solids:
  • At each vertex at least 3 faces meet


  • When you add up the internal angles that meet at a vertex, it must be less than 360 degrees.

  • Platonic Solid's faces are all identical regular polygons:

    A regular triangle has internal angles of 60°
    • 3 triangles (3×60°=180°)
    • 4 triangles (4×60°=240°)
    • or 5 triangles (5×60°=300°)
A square has internal angles of 90°, so there is only:
  • 3 squares (3×90°=270°)
A regular pentagon has internal angles of 108°, so there is only:
  • 3 pentagons (3×108°=324°)
At each vertex: Angles at Vertex
(Less than 360°)		 Solid  
3 triangles meet 180° tetrahedron
4 triangles meet 240° octahedron
5 triangles meet 300° icosahedron
3 squares meet 270° cube
3 pentagons meet 324° dodecahedron

Anything else has 360° or more at a vertex, which is impossible. Example: 4 regular pentagons (4×108° = 436°), 3 regular hexagons (3×120° = 360°), etc.





Platonic Solids for OpenSCAD - v0.7 was created by member WilliamAAdams. To use the files, download the following:
    1. maths geodesic.scad
    2. test platonic.scad
    3. platonic.scad
  2. Play around with the test_platonic.scad file to see how things are done
The 5 platonic solids:
  1. tetrahedron(rad=1)
  2. octahedron(rad=1)
  3. hexahedron(rad=1)
  4. dodecahedron(rad=1)
  5. icosahedron(rad=1)
are represented by functions that represent their geometry/topology, in a form suitable for rendering with the polyhedron() module 

//an icosahedron centered at [0,0,0], with a radius of 20.

display_polyhedron(icosahedron(20));
According to WilliamAAdams, "being able to set the radius is really handy as you can do things like nest them, or simply create them to the size you need. The fact that they're centered on the origin makes it fairly easy to rotate them around, to whatever orientation you like."



Here is another set of Platonic Solids created by member wouterglorieux:
Platonic solids set by wouterglorieux is licensed under the Creative Commons - Attribution - Share Alike license.